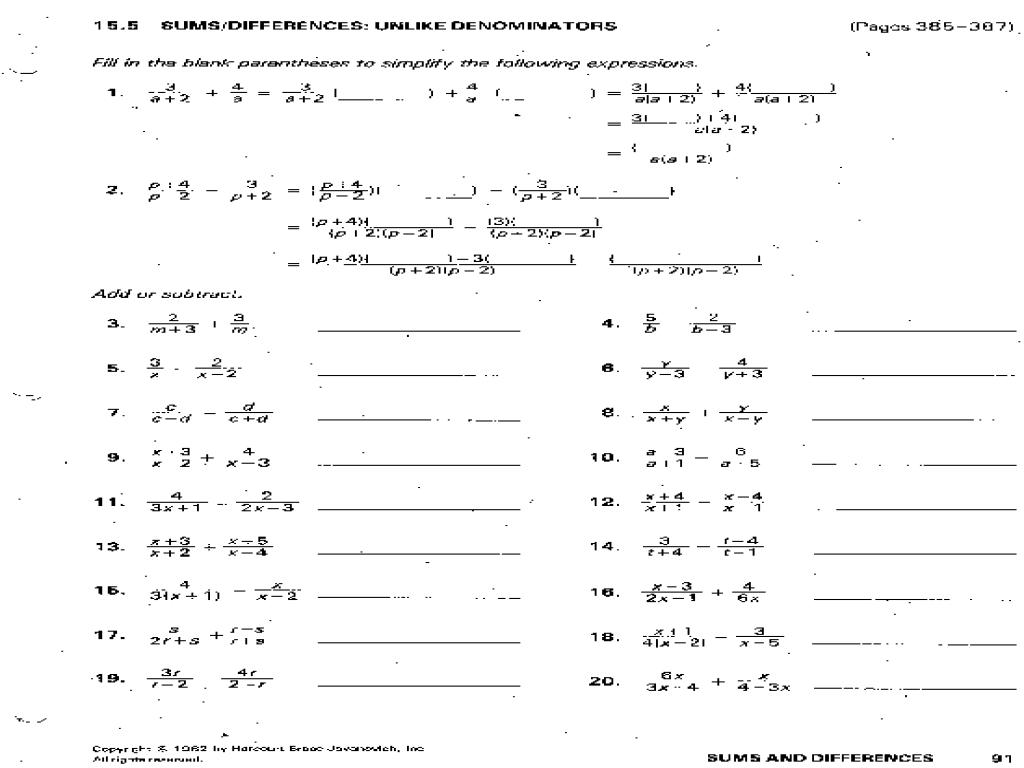
Adding and subtracting rational expressions worksheet answers – Embark on an enriching journey into the realm of rational expressions! This comprehensive guide, complete with a treasure trove of worksheet answers, unlocks the secrets of adding and subtracting these enigmatic mathematical entities. Prepare to witness the intricate dance of like and unlike denominators as we delve into the depths of rational expressions, revealing their practical applications in the tapestry of real-world scenarios.
Our voyage begins with an exploration of rational expressions with like denominators, where the harmonious union of these expressions yields elegant solutions. We shall then venture into the realm of unlike denominators, where the complexities of finding common denominators add a touch of intrigue to our quest.
Along the way, we shall encounter a plethora of examples, each illuminating the intricacies of these operations.
Adding and Subtracting Rational Expressions
Rational expressions are algebraic expressions that represent the quotient of two polynomials. Adding and subtracting rational expressions involves combining two or more rational expressions into a single expression.
Adding Rational Expressions with Like Denominators
When the denominators of two rational expressions are the same, we can add or subtract the numerators and keep the denominator.
Example:
(x + 2) / (x – 3) + (x – 1) / (x – 3) = (x + 2 + x – 1) / (x – 3) = (2x + 1) / (x – 3)
Subtracting Rational Expressions with Like Denominators, Adding and subtracting rational expressions worksheet answers
Similar to adding, when subtracting rational expressions with like denominators, we subtract the numerators and keep the denominator.
Example:
(x + 2) / (x – 3) – (x – 1) / (x – 3) = (x + 2 – x + 1) / (x – 3) = (3) / (x – 3)
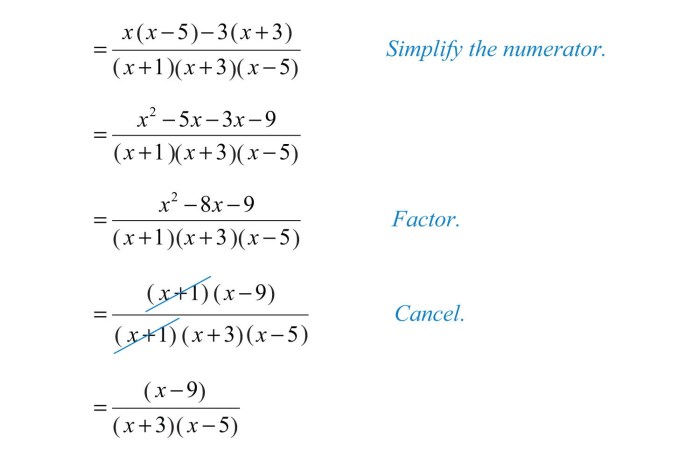
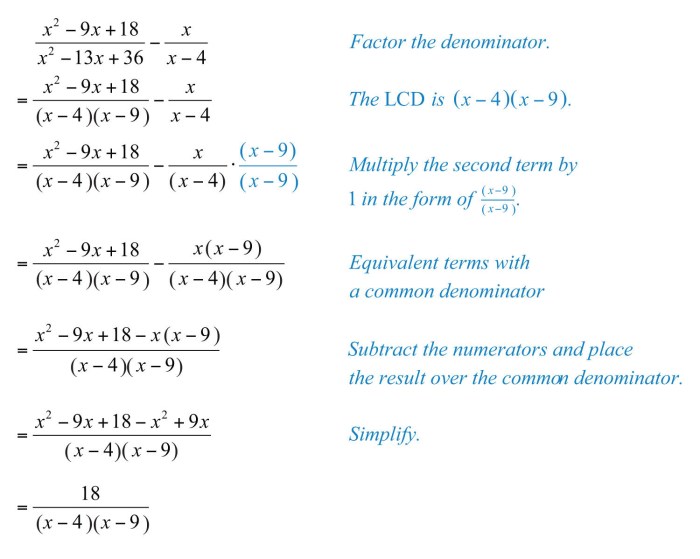
Adding and Subtracting Rational Expressions with Unlike Denominators
When the denominators of two rational expressions are different, we first need to find a common denominator before we can add or subtract them.
Example:
(x + 2) / (x – 3) + (x – 1) / (x + 2) = [(x + 2)(x + 2) + (x – 1)(x – 3)] / [(x – 3)(x + 2)] = (x 2+ 4x + 4 + x 2– 4x + 3) / (x 2– x – 6) = (2x 2+ 7) / (x 2– x – 6)
Applications of Adding and Subtracting Rational Expressions
Adding and subtracting rational expressions has various applications in different fields, including:
- Physics: Calculating velocity, acceleration, and force
- Chemistry: Determining concentrations and chemical reactions
- Engineering: Designing structures and systems
Popular Questions: Adding And Subtracting Rational Expressions Worksheet Answers
What is the key to adding rational expressions with like denominators?
The key lies in recognizing that the denominators remain unchanged, allowing us to focus solely on adding or subtracting the numerators.
How do we handle rational expressions with unlike denominators?
In such cases, we must first find a common denominator, which is the least common multiple of the original denominators. This enables us to rewrite the expressions with equivalent denominators, facilitating their addition or subtraction.